- #1
Martyn Arthur
- 84
- 16
- TL;DR Summary
- Area Integration
Hi I struggle with integration generally. Could you be able please to talk me through the stages of this one?
thanks
martyn
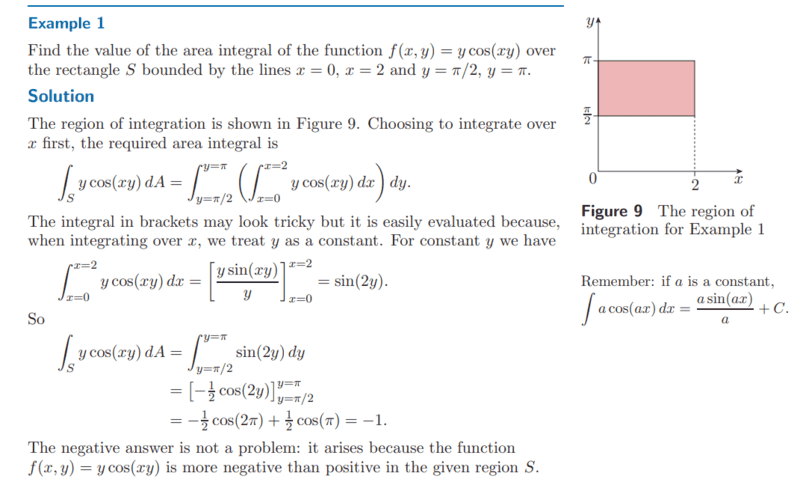
thanks
martyn
It would be helpful if you told us exactly which parts you don't understand. If your answer is "all parts," then my suggestion would be to go back to the start of the section in your textbook where iterated integration is introduced.Martyn Arthur said:Could you be able please to talk me through the stages of this one?
This integral is $$\int_{x=\pi/2}^\pi \sin(2y)~dy$$Martyn Arthur said:I am completely lost as to the overall mechanics of the conversion of sin to (-1/2cos).
Integral 2 is not the conclusion. It is the result of the integral in parentheses in integral 1.Martyn Arthur said:Given that integral 2 produces a conclusion how do they fit together.
I suspect at this level you are expected to recognise a common anti-derivative like this.Martyn Arthur said:I am completely lost as to the overall mechanics of the conversion of sin to (-1/2cos).
I can't make any sense of this of the attachments. They are the same image double-posted and are as follows:Martyn Arthur said:You guys are so patient. Given the following
xr^3(1-cos^2θ)cos^2θ
I need to make the substitution u = (1-cos^2θ) have I got it right?
Thanks
View attachment 340095